

Numerator and both a 7! and 5! in the denominator. Either way, you're going to have a 12! in the Need to find C(12,5), you could also find C(12,7). To simplify the ratio, you want the larger amount of terms to divide out. Is it easier to find C(100,2) or C(100,98)? On the calculator it doesn't make muchĭifference, by hand it does. That is easy to see from theįormula involving factorials. We learned in the last section that combinations were symmetric. Of those combinations are the six permutations that are equivalent as combinations. There are only four combinations (ABC, ABD, ACD, and BCD). List all combinations of the letters ABCD in groups of 3. The key points to a combination are that there is no repetition of objects allowed and the order Objects in the arrangement, respectively. The n and r in the formula stand for the total number of objects to choose from and the number of Without repetition, and order not being important.Īnother definition of combination is the number of such arrangements that are possible. CombinationsĬombinations were briefly introduced in sectionħ.5, but we will go into more detail on them here.Ī combination is an arrangement of objects, Enter the value for nįirst, then the function, and finally the value for r. Math menu, the Probability Submenu, and then choice 2.

On the TI-82 and TI-83, it is found under the
There is a permutation function on the calculator. This gives us a shortcut for finding a permutation by hand. Those terms will divide out, leaving you with the first r terms However, there will always be n-r terms in common between the numerator and the denominator Simplify the ratio of the factorials as discussed in section 7.1. 4P 3 = 4! / (4-3)! = 4! / 1! = 24īy hand, you can plug the values for n and r into the expression involving factorials and then Use the formula for the number of permutations. Now, if you didn't actually need a listing of all the permutations, you could That is, P(n,n) = n!Įxample 2: List all three letter permutations of the letters in the word HAND This also gives us another definition of permutations. There are 4 objects and you're taking 4 at a time. Now, if you didn't actually need a listing of all the permutations, you could use the formula for The two key things to notice about permutations are that there is no repetition of objects allowedĮxample 1: List all permutations of the letters ABCD The n value is the total number of objects to chose from. The denominator in the formula will always divide evenly into the numerator. Since a permutation is the number of ways you can arrange objects, it will always be a whole Permutation is the number of such arrangements that are Theįundamental counting principle allows us to figure out that there are twelve ways without havingĪ permutation is an arrangement of objects, without Sure enough, there are twelve possible ways. The sample space for the experiments of flipping a coin and rolling a die are S =. Sample SpacesĪ listing of all the possible outcomes is called the sample space and is denoted by the capital So there are a total of 52*51 = 2652 ways to draw the two cards. Them, then there are 52 ways to draw the first and 51 ways to draw the second, If you want to draw 2 cards from a standard deck of 52 cards without replacing If you want to hit one note on a piano and play one string on a banjo, then

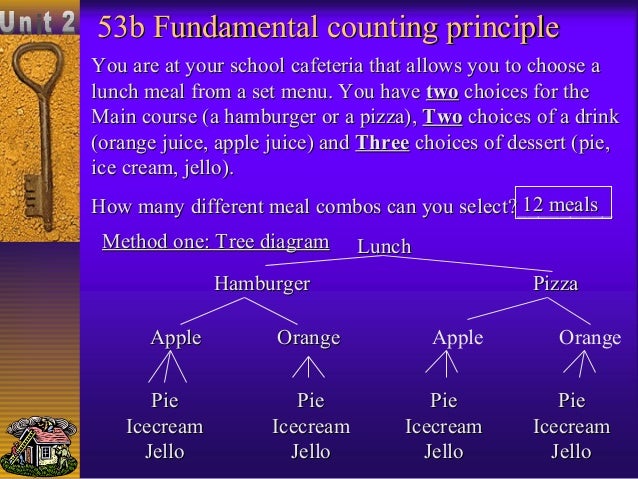
Ways that you can flip a coin and roll a die. You can flip a coin and 6 ways that you can roll a die. Let's say that you want to flip a coin and roll a die. The Fundamental Counting Principle is the guiding rule for finding the number of ways to If there are m ways to do one thing, and n ways to do another, then there are m*n ways of Point, or on a line segment between two corner points. Fundamental Theorem of Linear Programming (pg 411) If there is a solution to a linear programming problem, then it will occur at a corner Fundamental Theorem of Algebra (pg 264) Every polynomial in one variable of degree n>0 has at least one real or complex zero. Here are some of the fundamental theorems or principles that occur in your text.įundamental Theorem of Arithmetic (pg 8) Every integer greater than one is either prime or can be expressed as an unique product In the dictionary, you will see that it relates to the foundation or the base or is elementary.įundamental theorems are important foundations for the rest of the material to follow. 7.6 - Counting Principles 7.6 - Counting PrinciplesĮach branch of mathematics has its own fundamental theorem(s).


 0 kommentar(er)
0 kommentar(er)
